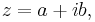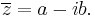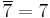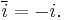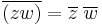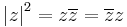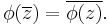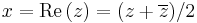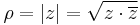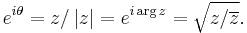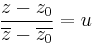Complex conjugate

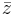Geometric representation of z and its conjugate \bar{z} in the complex plane

In mathematics, complex conjugates are a pair of complex numbers, both having the same real part, but with imaginary parts of opposite signs.[1][2] For example, 3 + 4i and 3 − 4i are complex conjugates.

The conjugate of the complex number 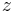z

z=a+ib,\,

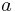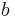where a and b are real numbers, is

\overline{z} = a - ib.\,

For example,

\overline{(3-2i)} = 3 + 2i
\overline{7}=7
\overline{i} = -i.

An alternate notation for the complex conjugate is z*. However, the \bar z notation avoids confusion with the notation for the conjugate transpose of a matrix, which can be thought of as a generalization of complex conjugation. If a complex number is represented as a 2×2 matrix, the notations are identical.

Complex numbers are often depicted as points in a plane, which is a variation of the Cartesian coordinate system (see diagram). The 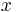x-axis contains the real numbers and the 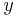y-axis contains the multiples of 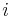i. In this view, complex conjugation corresponds to reflection at the x-axis.

In polar form, the conjugate of r e^{i \phi} is r e^{-i \phi}. Euler's formula confirms this.

Pairs of complex conjugates are significant because the imaginary unit 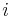i is qualitatively indistinct from its additive and multiplicative inverse 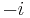-i, as they both satisfy the definition for the imaginary unit: 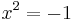x^2=-1. Thus in most "natural" settings, if a complex number provides a solution to a problem, so does its conjugate, such as is the case for complex solutions of the quadratic formula with real coefficients.

Contents

Properties

These properties apply for all complex numbers z and w, unless stated otherwise, and can be easily proven by writing z and w in the form a + ib.

\overline{(z + w)} = \overline{z} + \overline{w} \!\
\overline{(zw)} = \overline{z}\; \overline{w} \!\
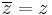\overline{z} = z \!\ if and only if z is real
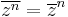\overline{z^n} = \overline{z}^n for any integer n
\left| \overline{z} \right| = \left| z \right|
{\left| z \right|}^2 = z\overline{z} = \overline{z}z
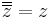\overline{\overline{z}} = z \!\ , involution (i.e., the conjugate of the conjugate of a complex number z is again that number)
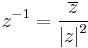z^{-1} = \frac{\overline{z}}{{\left| z \right|}^2} if z is non-zero

The latter formula is the method of choice to compute the inverse of a complex number if it is given in rectangular coordinates.

\exp(\overline{z}) = \overline{\exp(z)}\,\!
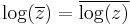\log(\overline{z}) = \overline{\log(z)}\,\! if z is non-zero

In general, if 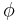\phi\, is a holomorphic function whose restriction to the real numbers is real-valued, and \phi(z)\, is defined, then

\phi(\overline{z}) = \overline{\phi(z)}.\,\!

Consequently, if 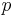p is a polynomial with real coefficients, and 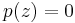p(z) = 0, then 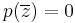p(\overline{z}) = 0 as well. Thus, non-real roots of real polynomials occur in complex conjugate pairs (see Complex conjugate root theorem).

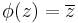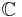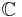The function \phi(z) = \overline{z} from \mathbb{C} to \mathbb{C} is a homeomorphism (where the topology on 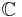\mathbb{C} is taken to be the standard topology). Even though it appears to be a well-behaved function, it is not holomorphic; it reverses orientation whereas holomorphic functions locally preserve orientation. It is bijective and compatible with the arithmetical operations, and hence is a field automorphism. As it keeps the real numbers fixed, it is an element of the Galois group of the field extension \mathbb{C}/\mathbb{R}. 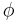This Galois group has only two elements: \phi and the identity on \mathbb{C}. Thus the only two field automorphisms of \mathbb{C} that leave the real numbers fixed are the identity map and complex conjugation.

Use as a variable

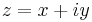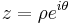Once a complex number z = x + iy or z = \rho e^{i\theta} is given, its conjugate is sufficient to reproduce the parts of the z-variable:

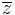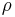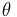Thus the pair of variables z\, and \overline{z} also serve up the plane as do x,y and \rho \, and \theta. Furthermore, the 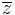\overline{z} variable is useful in specifying lines in the plane:

\{z \ :\ z \overline{r} + \overline{z} r = 0 \}

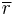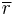is a line through the origin and perpendicular to \overline{r} since the real part of z\cdot\overline{r} is zero only when the cosine of the angle between z\, and \overline{r} is zero. Similarly, for a fixed complex unit u = exp(b i), the equation:

\frac{z - z_0}{\overline{z} - \overline{z_0}} = u

determines the line through z_0\, in the direction of u.

These uses of the conjugate of z as a variable are illustrated in Frank Morley's book Inversive Geometry (1933), written with his son Frank Vigor Morley.

Generalizations

The other planar real algebras, dual numbers, and split-complex numbers are also explicated by use of complex conjugation.

Taking the conjugate transpose (or adjoint) of complex matrices generalizes complex conjugation. Even more general is the concept of adjoint operator for operators on (possibly infinite-dimensional) complex Hilbert spaces. All this is subsumed by the *-operations of C*-algebras.

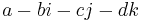One may also define a conjugation for quaternions and coquaternions: the conjugate of a + bi + cj + dk is a - bi - cj - dk.

Note that all these generalizations are multiplicative only if the factors are reversed:

{\left(zw\right)}^* = w^* z^*.

Since the multiplication of planar real algebras is commutative, this reversal is not needed there.

There is also an abstract notion of conjugation for vector spaces 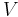V over the complex numbers. In this context, any (real) linear transformation 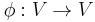\phi: V \rightarrow V that satisfies

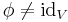  1. \phi\neq \operatorname{id}_V, the identity function on V,
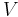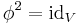  2. \phi^2 = \operatorname{id}_V\,, and
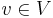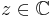  3. \phi(zv) = \overline{z} \phi(v) for all v\in V, z\in{\mathbb C},

is called a complex conjugation. One example of this notion is the conjugate transpose operation of complex matrices defined above. It should be remarked that on general complex vector spaces there is no canonical notion of complex conjugation.

See also

References

  1. ↑ Weisstein, Eric W., "Complex Conjugates" from MathWorld.
  2. ↑ Weisstein, Eric W., "Imaginary Numbers" from MathWorld.